Abstract
The investigation indicates an experimental study on the convective heat transfer of Cu/Ethylene Glycol nanofluid flow inside a concentric annular tube with constant heat flux boundary condition and proposes a novel correlation for the prediction of Nusselt number. For extending the previous study by Jafarimoghaddam et al., we selected the nanoparticles with the average size of 20 nm and also other conditions of the experiment are based on Jafarimoghaddam et al. (2016) [1]. The applied nanofluid was prepared by Electrical Explosion of Wire technique with no accumulation during the experiment. The tube was heated using an electrical heating coil covered it. The effects of different parameters such as flow Reynolds number and nanofluid particle concentration on heat transfer coefficient are studied. The acquired experimental data were used to establish a correlation for predicting Nusselt number of nanofluid flow inside the annular tube. This correlation has been presented by using the exponential regression analysis and least square method. Correlation is valid for Cu/Base Ethylene Glycol nanofluid flow with the volume concentrations between 0.011 and 0.171 in the hydrodynamically fully developed laminar flow regime with Re < 160 which is most applicable in micro heat sinks.
Keywords
Cu/Ethylene Glycol nanofluid; Annular tube; Laminar flow; Constant heat flux boundary condition
Nomenclatures
Symbol- Description (unit)
Re- Reynolds number (–)
Cp- specific heat capacity (J/kg K)
Nunf- nanofluid Nusselt number (–)
Pr- Prandtl number (–)
- volume fraction (–)
- mass rate (kg/s)
h- convective heat transfer coefficient (W/m2 K)
f- friction factor (–)
Pe- Peclet number (–)
K- thermal conductivity (W/m K)
T- temperature (K)
D- diameter (m)
- density (kg/m3)
- heat flux (W)
- velocity (m/s)
- pressure drop (Pa)
μ- viscosity (N s/m2)
x- distance (m)
1. Introduction
Impact and effectiveness of heat transfer on equipment of the industries, on the one hand, and the costly and limited ways to increase the heat transfer (e.g. expansion of the heat transfer surface) on the other hand, lead to an urgent need for alternative solutions to increase the heat transfer process such as using Nanofluids. Nanofluid technology offers high potential for the development of cooling systems with high performance, in small size and economical consideration. Xuan and Roetzel [2] proposed an approach for deriving heat transfer correlation of the Nanofluid as follows:
|
|
(1) |
Here and are the derivatives of the dimensionless velocity and the dimensionless temperature, respectively. They proposed that C∗ was a constant to be determined from the experiment and the effects of transport properties of the Nanofluid and thermal dispersion were included. Vajjha [3] developed new correlation for convective heat transfer in turbulent flow regime for Nanofluids. They indicated that heat transfer coefficient of nanofluids showed an increase with the particle volumetric concentration and the pressure loss of nanofluids also increases with an increase in particle volume concentration. Their new equation is a function of the nanoparticle volume concentration in addition to the Reynolds number and the Prandtl number:
|
|
(2) |
Above equation has maximum deviations of ±10% and an average deviation of 2% when compared with the experimental data points. The correlation is valid for 3000 < Re < 16,000, 0 < φ < 0.06 for CuO and SiO2 Nanofluids and 0 < φ < 0.1 for the Al2O3 Nanofluid. Xuan and Li [4] conducted the experiments with Cu base water nanofluid and obtained a convective heat transfer equation for nanofluids under the turbulent flow condition as follows:
|
|
(3) |
Proposed correlation was limited to a 2% volume concentration. Lai et al. [5] reported enhancement of Nusselt number about 8% for Al2O3-water nanofluid with nanoparticle volume fraction of 1% and size of 20 nm when the flow regime was laminar.
Ben Mansour et al. [6] experimentally investigated mixed convection of Al2O3 water Nanofluid inside an inclined copper tube submitted to a uniform wall heat flux at its outer surface and proposed two new correlations to calculate the Nusselt number in the fully developed region for horizontal and vertical tubes, for Rayleigh number from 5 × 105 to 9.6 × 105, Reynolds number from 350 to 900 and particle volume concentrations up to 4%.
Asirvatham et al. [7] performed an experimental investigation on convective heat transfer of Nanofluids using silver–water Nanofluids under laminar, transition and turbulent flow regimes in a horizontal 4.3 mm inner-diameter tube-in-tube counter-current heat transfer test section. Based on the experimental data and calculated results, they presented a new correlation could take into account the main factors that affect heat transfer of the Nanofluid and could be used to predict heat transfer coefficient of Nanofluids with ±10% deviation.
As an example of new Nanofluids an experimental work entitled by: “A fully developed laminar convective heat transfer and pressure drop characteristics through a uniformly heated circular tube using Al2O3–Cu/water hybrid nanofluid” has been presented by Suresh et al. [8]. Their results indicated a maximum enhancement of 13.56% in Nusselt number at a Reynolds number of 1730 when compared to Nusselt number of water. The empirical correlation proposed for Nusselt number, was in good agreement with the experimental data. The regression equation coefficients were assessed with the help of classical least square method and the correlation is valid for laminar flow with Re < 2300, dilute Al2O3–Cu/water hybrid nanofluid with volume concentration φ = 0.1%.
Razi et al. [9] investigated pressure drop and thermal characteristics of CuO–oil nanofluid in laminar flow regime in flattened tubes with the heights of 11.5 mm (as round tube), 9.6 mm, 8.3 mm, 7.5 mm and 6.3 mm under constant heat flux boundary condition. Their results showed that the heat transfer performance is improved as the tube profile is flattened. Flattening the tube profile resulted in pressure drop increasing. Also they showed that, Nanofluids have better heat transfer characteristics when they flow in flattened tubes rather than in the round tube. Compared to pure oil flow, maximum heat transfer enhancement of 16.8%, 20.5% and 26.4% is obtained for Nanofluid flow with 2 wt.% concentration inside the round tube and flattened tubes with internal heights of 8.3 and 6.3 mm, respectively. In addition a correlation was developed to predict the nanofluid flow heat transfer coefficient inside the flattened tubes. This correlation predicts the experimental data within an error band of ±20%.
Moreover, Aberoumand et al. and Abbasian Arani et al. have presented a comprehensive study on any oil-based nanofluids [10], [11] and [12] but because of the lack of experimental works on annular tubes and Ethylene Glycol as base fluid in comparison with other reported studies in literature, and this experimental study is done and a correlation to predict Nusselt number in different volume fraction and Reynolds number was developed. The aim of this study was the empirical investigation of heat transfer in concentric annular tube at low concentration in laminar flow. The investigation was started by preparing the nanofluid. Thermal conductivity and dynamics viscosity were measured before using the nanofluid in experimental setup. The temperature across the tube was measured during the experimental test for steady state condition with constant heat flux condition. The average friction factor and thermal heat transfer coefficient were then evaluated. In each section a complete discussion about obtaining results was presented.
2. Second section
2.1. Nanofluids preparation
Electrical Explosion Wire (E.E.W) as a one step method has been used. In this method by applying extra high electric voltage and current, the primary bulk wire is converted into the nanoparticles via pulse explosive process. It is necessary to explain that, another special feature of this system is the possibility of adding a surfactant to the liquid. So, the nanofluid produced with this method, saves primary distribution for a long time. Among all of the existing methods in the production of metal nanoparticles, electrical explosion method is the most economical and industrial method [13].
2.2. Experimental apparatus
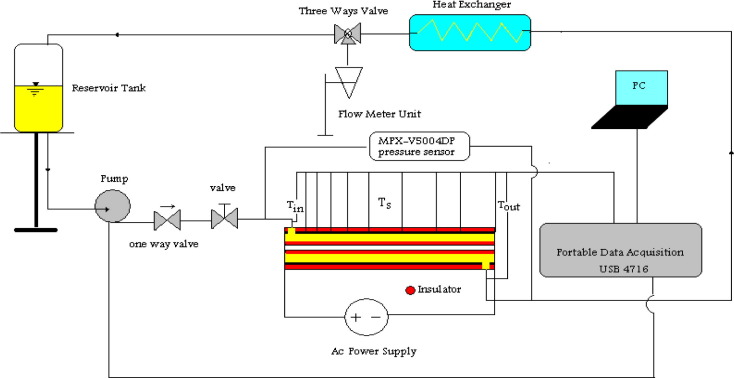
The experimental setup chiefly includes a flow loop, including a pump, a nanofluid reservoir, a gate valve, a non return valve, test section, RTD PT100 temperature sensors, MPX-V5004DP pressure sensor, data analyzer USB 4716 and a three way valve. Test section has built of two copper tubes. Inner tube has 1500 mm length and its inner diameter is 6.35 mm and 1.0 mm thickness. Outer tube has 1500 mm length and 25.4 mm inner diameter and 1.3 mm thickness. An electrical wire coil wrapped around the outer copper tube, which links to AC power supply. Two calibrated temperature RTD PT100 sensors are installed in entrance and exit the test section for measurement inlet temperature and outlet temperature of fluids. Nine RTD PT100 sensors were employed to measure the wall temperature of the test section and all of them welded optionally at 3, 7, 15, 25, 45, 100, 120, 130, 150 cm of axial distance. A 1 litter vessel and stopwatch accurate to 0.001 s were used to measure the flow rate. Heat exchanger unit settle in after the test section. Fluid turns upside down to the pump from the fluid reservoir, then it pumps to the test section and bulk temperatures and wall temperatures are measured with sensors.
3. Data analysis
3.1. Data collection
To determine the specific heat capacity (cp) in different temperature of nanofluids and pure Ethylene Glycol, a differential scanning calorimeter (DSC F3 Maia, manufactured by NETZSCH-Germany) was used. SVM3000 device was used to determine the density in different temperatures and weight fractions of nanofluid. The thermal conductivity and viscosity of nanofluid and pure Ethylene Glycol were measured by KD2 thermal properties analyzer and Brookfield viscometer (DV-II+Pro Programmable Viscometer), respectively (see Fig. 1 and Table 1 and Table 2).
|
|
|
Figure 1. Schematic of experimental setup.
|
| No. | φ (%) | H (W/m2 K) | Nu | Re | Pr | Q (cm3/s) | No. | φ (%) | H (W/m2 K) | Nu | Re | Pr | Q (cm3/s) |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| 1 | 0 | 79.98 | 13.34 | 26.6 | 402.6 | 10 | 26 | 0.044 | 99.7 | 15.99 | 60.03 | 437.2 | 30 |
| 2 | 0 | 85.01 | 14.13 | 37.9 | 411.3 | 15 | 27 | 0.044 | 107.9 | 17.87 | 63.54 | 438.9 | 34 |
| 3 | 0 | 91.00 | 14.58 | 50.9 | 421.2 | 20 | 28 | 0.044 | 112.7 | 18.47 | 69.9 | 451.5 | 39 |
| 4 | 0 | 98.01 | 15.33 | 57.7 | 435.3 | 25 | 29 | 0.044 | 118.8 | 19.75 | 85.7 | 452.4 | 42 |
| 5 | 0 | 100.0 | 16.02 | 62.8 | 442.1 | 33 | 30 | 0.044 | 125.2 | 20.89 | 87.7 | 453.9 | 48 |
| 6 | 0 | 103.4 | 16.44 | 69.9 | 446.5 | 38 | 31 | 0.044 | 135.5 | 25.38 | 97.17 | 454.4 | 52 |
| 7 | 0 | 105.1 | 16.95 | 87.7 | 448.0 | 48 | 32 | 0.044 | 139.3 | 27.03 | 100.9 | 458.8 | 58 |
| 8 | 0 | 107.8 | 17.22 | 100.9 | 449.5 | 58 | 33 | 0.044 | 142.7 | 28.1 | 123.1 | 458.9 | 65 |
| 9 | 0 | 110.7 | 17.98 | 127.9 | 450.9 | 68 | 34 | 0.044 | 145.9 | 28.89 | 129.9 | 461.1 | 71 |
| 10 | 0.022 | 82.30 | 13.6 | 26.6 | 410.7 | 10 | 35 | 0.044 | 148.2 | 29.18 | 139.9 | 464.4 | 78 |
| 11 | 0.022 | 88.24 | 14.8 | 37.9 | 419.1 | 15 | 36 | 0.044 | 150.1 | 29.61 | 148.8 | 465.1 | 83 |
| 12 | 0.022 | 97.93 | 14.9 | 50.8 | 435.1 | 19 | 37 | 0.171 | 113.28 | 24.12 | 26.6 | 428.7 | 10 |
| 13 | 0.022 | 100.9 | 15.7 | 56.6 | 444.0 | 23 | 38 | 0.171 | 115.79 | 26.24 | 39 | 438.6 | 16 |
| 14 | 0.022 | 106.1 | 17.8 | 59.61 | 449.3 | 29 | 39 | 0.171 | 129.88 | 27.81 | 50.9 | 443.5 | 20 |
| 15 | 0.022 | 110.0 | 18.2 | 62.8 | 450.0 | 33 | 40 | 0.171 | 133.91 | 31.91 | 57.7 | 447.2 | 25 |
| 16 | 0.022 | 115.8 | 19.1 | 70.2 | 451.3 | 39 | 41 | 0.171 | 144.13 | 32.21 | 60.1 | 448.2 | 30 |
| 17 | 0.022 | 118.4 | 19.9 | 85.7 | 452.1 | 42 | 42 | 0.171 | 147.10 | 33.12 | 64.5 | 449.3 | 35 |
| 18 | 0.022 | 119.8 | 20.3 | 100.2 | 452.9 | 56 | 43 | 0.171 | 149.73 | 33.59 | 72.4 | 451.6 | 40 |
| 19 | 0.022 | 121.8 | 20.4 | 118.8 | 453.1 | 63 | 44 | 0.171 | 151.1 | 33.95 | 83.5 | 452.5 | 45 |
| 20 | 0.022 | 128.1 | 21.5 | 129.5 | 453.9 | 70 | 45 | 0.171 | 163.3 | 34.17 | 90.19 | 452.1 | 50 |
| 21 | 0.022 | 129.0 | 21.94 | 130.4 | 454.1 | 72 | 46 | 0.171 | 165.6 | 34.22 | 99.71 | 452.9 | 55 |
| 22 | 0.044 | 86.14 | 13.89 | 26.6 | 418.0 | 10 | 47 | 0.171 | 179.4 | 35.75 | 123.1 | 453.6 | 65 |
| 23 | 0.044 | 92.52 | 14.5 | 37.9 | 428.1 | 15 | 48 | 0.171 | 181.3 | 36.13 | 134.2 | 454.5 | 75 |
| 24 | 0.044 | 94.15 | 15.12 | 50 | 433.8 | 21 | 49 | 0.171 | 182.8 | 37.54 | 151.39 | 454.3 | 85 |
| 25 | 0.044 | 97.52 | 15.9 | 58.52 | 435.6 | 26 | 50 | 0.171 | 183.9 | 37.77 | 160.0 | 455.3 | 95 |
| m | 1 | 2 | 3 | 4 | 5 |
|---|---|---|---|---|---|
| 0.007630 | 0.002053 | 0.000903 | 0.000491 | 0.000307 | |
| 28.87 | 89.3 | 204.3 | 326.5 | 530.9 |
With the consideration of the energy balance on a differential control volume of the fluid inside the tube [14]:
|
|
(4) |
where and P are mass flow rate and perimeter of the tube, respectively. Ts, Tb and cp are the surface temperature, bulk temperature and specific heat capacity respectively. x is distance from the tube inlet. The convective heat transfer coefficient was calculated as follows:
|
|
(5) |
where Do and Di are outer diameter and inner diameter respectively. Reynolds, Prandtl number, Peclet number and friction factor are defined as follows:
|
|
(6) |
3.2. Uncertainty
All parameters used in the experimental study for calculating the major heat transfer characteristics such as Nusselt number, convective heat transfer coefficient and friction factor have uncertainties due to inherent errors. In this work, Coleman and Steele uncertainty method [15] was employed for this purpose. The maximum uncertainties corresponding to the Nusselt number, convective heat transfer coefficient and friction factor are 7.4%, 3.3% and 8.1% respectively. The uncertainty in experimental study was reported other researcher were about 2.45–5% for Nusselt number.
4. Result and discussion
4.1. Validation check
In order to verify the correctness of the experimental setup, initial experiments are performed with Ethylene Glycol as the working fluid. Because of low Reynolds number and high Prandtl number of Ethylene Glycol, hydrodynamically fully developed laminar flow and thermal entrance region for flow, are assumed respectively, for theoretical calculations (xt/D < 0.05RePr). The values of Nusselt numbers that are measured experimentally are compared with the values obtained by the following theoretical equation presented in Eq. (7)[14]:
|
|
(7) |
In this coefficient, μs is calculated at the surface temperature, while μm is evaluated at the bulk temperature. This solution is used for obtaining local Nusselt number of a fluid flow with temperature varying viscosity inside round tube under constant heat flux condition. Having the local Nusselt numbers at nine axial locations the average Nusselt numbers are obtained. Table 3 shows the values of experimental data and predicted theoretical values and relative errors between them.
| Exp. | 15.10 | 16.53 | 18.11 | 19.16 | 19.96 | 23.90 | 24.41 | 24.99 | 27.30 | 28.10 | 29.26 | 31.53 | 33.75 |
| The. | 15.84 | 16.79 | 18.20 | 19.38 | 20.12 | 24.01 | 24.31 | 24.73 | 24.39 | 28.89 | 29.02 | 31.20 | 33.50 |
| Err. | 4.67% | 1.55% | 0.49% | 1.1% | 0.8% | 0.46% | 0.4% | 1.0% | 3.1% | 2.7% | 0.7% | 1.% | 0.8% |
4.2. Heat transfer characteristics
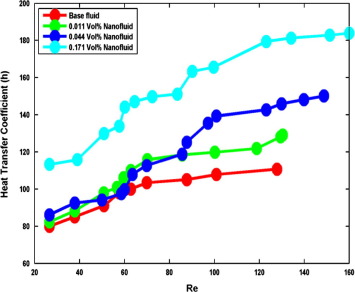
Variation of heat transfer coefficient related to Cu- Ethylene Glycol nanofluid as a function of Reynolds number for pure Ethylene Glycol and the nanofluid with 0.011, 0.044 and 0.171% volume fraction flow inside concentric annulus tube at constant heat fluxes of 204 watt is shown in Fig. 2. Based on the results, for certain Reynolds number, the convective heat transfer coefficient (h) of nanoparticles suspended in base Ethylene Glycol is higher than that in base Ethylene Glycol. This enhancement noticeably is reliant on the concentration of nanoparticles.
|
|
|
Figure 2. Variation of mean heat transfer coefficient versus Reynolds number in concentric annular tube.
|
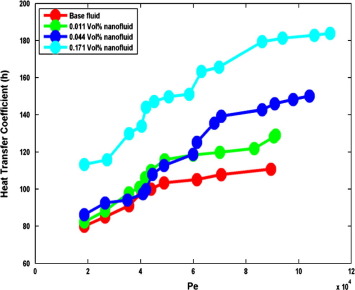
Fig. 3 shows the results for Cu- Ethylene Glycol nanofluid at various Peclet numbers at 204 watt on outer tube and laminar flow in concentric annulus tube. It shows the increments in heat transfer coefficient by increasing the Peclet number. The enhancement of Nusselt number and heat transfer coefficient exhibit that the heat transfer performance of nanofluid relies on various parameters such as nanoparticles movement, Brownian motion, reduction in thermal boundary layer thickness and possible slip condition at the walls which are the possible reasons for enhancement of heat transfer coefficient respectively, and the thermal conductivity is not the only main factor. It may be noted that the improvement in the heat transfer coefficient is much higher compared to the increase in thermal conductivity. This conclusion has been referred by other researchers such as Farajollahi et al. [16].
|
|
|
Figure 3. Variation of mean heat transfer coefficient versus Peclet number in concentric annular tube.
|
The ratio of convective heat transfer coefficient of Cu- Ethylene Glycol nanofluid to the base fluid, versus Reynolds number was measured in this study. Results indicated that the average heat transfer coefficient in 0.011, 0.044 and 0.171% volume fraction increases approximately 9.3%, 21.12% and 31.1% respectively.
The effects of volume concentration and nanofluid Reynolds number may be explained by the macroscopic theory for the forced convective heat transfer. Based on this theory the convective heat transfer coefficient, may be formulated approximately as h = kf/dt, in which kf is thermal conductivity and dt is thermal boundary layer. Both an increase in kf or/and a decrease in dt enhance the convective heat transfer coefficient. As explained previously increase in Reynolds number leads to a decrease in the boundary layer thickness. Also an addition of nanoparticles to base fluid increases the thermal conductivity of nanofluid and the enhancement increases with increasing particle concentration. The increase of the thermal conductivity should increase the convective heat transfer coefficient. However, the increase in particle concentration also increases the fluid viscosity, which should result in an increase in the boundary layer thickness hence a decrease in the convective heat transfer coefficient. As shown clearly in this study, addition of nanoparticles enhances the convective heat transfer. These results demonstrate that the positive effect of the thermal conductivity enhancement overcomes the negative effect of the viscosity increase under the conditions of this investigation. It is worth to note that a large number of researchers believe that particle size has a marginal effect (compared to Reynolds number and volume concentration) on the convective heat transfer coefficient under the conditions of this work. This concept comes from the reality that nanofluids containing larger nanoparticles have a lower thermal conductivity and a higher viscosity, both of two effects should have led to a lower convective heat transfer coefficient. Wen and Ding [17] related this effect to the particle migration mechanism. According to this idea, large nanoparticles tend to migrate to the central part of the pipe, which could result to a particle depletion region with low viscosity at the near wall hence a decrease in the boundary layer thickness. Also, small nanoparticles tend to be uniformly distributed over the pipe cross-section due to the Brownian motion. Hence, for a given average particle concentration, the wall region could have a higher solids concentration and hence a higher viscosity when the flowing nanofluids contain smaller nanoparticles. The combination of the above two opposite cited effects could have been the consequence for the observed marginal effect of nanoparticle size under the conditions of this investigation. It must be cited that, the proposed particle migration mechanism is a hypothesis; further experimental study is needed for the verification and confirmation of this conclusion.
Using the present experimental data, the following correlations are developed to predict Nusselt number and convective heat transfer coefficient of nanofluid flow inside the annular tubes using nonlinear regression analysis. These correlations are valid for Cu/Base Oil nanofluid flow with weight concentrations 0.12, 0.36 and 0.72 in the hydrodynamically fully developed laminar flow regime with Re < 160, Di/Do = 0.25 and P = 204 W.
|
|
(8) |
This equation predicts the Nusselt Number falling within ±10% of the acquired experimental values.
The ratio of friction factor of Cu- Ethylene Glycol nanofluid to the base fluid versus velocity was quantified in the present work. Results show the average ratio of (fnf/fbf) was about 1.002, 1.009 and 1.014 for 0.011, 0.044 and 0.171% volume fraction. Therefore, there is no significant increase in friction factor for nanofluids.
5. Conclusion
Electrical Explosion Wire (E.E.W) as a one step method with special characteristics such as low cost, high efficiency and environmental friendly has been used for producing the Cu- Ethylene Glycol nanofluids. The values of heat transfer coefficient of base fluid and Cu- Ethylene Glycol nanofluid as a function of Reynolds number at 0.011, 0.044 and 0.171% volume fraction for flow inside concentric annular tube at constant heat fluxes boundary condition in laminar flow regime were measured. Based on these results, for all Reynolds number and value of nanoparticle concentration under study the convective heat transfer coefficient (h) of nanofluids was higher than base fluid. For higher volume fraction higher heat transfer coefficient was obtained. Moreover results indicated that the average heat transfer coefficient in 0.011, 0.044 and 0.171% volume fraction increases about 9.3%, 21.12% and 31.1% respectively. This investigation showed that there is non-significant increase in pressure drop of nanofluid with the increase in particle concentration compared to base fluid. A correlation was developed to predict the nanofluid flow Nusselt Number inside the concentric annular tubes. This correlation predicts the present experimental data within an error band of ±10% which can be considered for micro heat sinks applications.
References
- [1] A. Jafarimoghaddam, S. Aberoumand, H. Aberoumand; Experimental study on CU/OIL nanofluids through concentric annular tube: a correlation – Heat Transfer- Asian Research; John Wiley & Sons Publication (2016) http://dx.doi.org/10.1002/htj.21210
- [2] Y. Xuan, W. Roetzel; Conception for heat transfer correlation of nanofluids; Int. J. Heat Mass Transfer, 43 (2000), pp. 3701–3707
- [3] R.S. Vajjha, D.K. Das, D.P. Kulkarni; Development of new correlations for convective heat transfer and friction factor in turbulent regime for nanofluids; Int. J. Heat Mass Transfer, 53 (2010), pp. 4607–4618
- [4] Y. Xuan, Q. Li; Investigation on convective heat transfer and flow features of nanofluids; J. Heat Transfer, 125 (2003), pp. 151–155
- [5] W.Y. Lai, B. Duculescu, P.E. Phelan, R.S. Prasher; Convective heat transfer with nanofluids in a single 1.02-mm tube; Proceedings of ASME International Mechanical Engineering Congress and Exposition (IMECE) (2006), pp. 65–76
- [6] R. Ben Mansour, N. Galanis, C.T. Nguyen; Experimental study of mixed convection with watereAl2O3 nanofluid in inclined tube with uniform wall heat flux; Int. J. Therm. Sci., 50 (2011), pp. 403–410
- [7] L.G. Asirvatham, B. Raja, D.M. Lai, S. Wongwises; Convective heat transfer of nanofluids with correlations; Particuology, 9 (2011), pp. 626–631
- [8] S. Suresh, K.P. Venkitaraj, P. Selvakumar, M. Chandrasekar; Effect of Al2O3–Cu/water hybrid nanofluid in heat transfer; Exp. Therm. Fluid Sci., 38 (2012), pp. 54–60
- [9] P. Razi, M.A. Akhavan-Behabadi, M. Saeedinia; Pressure drop and thermal characteristics of CuO–base oil nanofluid laminar flow in flattened tubes under constant heat flux; Int. Commun. Heat Mass Transfer, 38 (2011), pp. 964–971
- [10] Sadegh Aberoumand, Kourosh Javaherdeh, Amin Jafarimoghaddam, Hossein Aberoumand; Synthesis and Rheological Behavior of Novel Ecofriendly Ag–Oil Nanofluid and Introduce General Correlations for Thermal Conductivity and Viscosity of Any Oil-Based Nanofluids, Heat Transfer – Asian Research, John Wiley & Sons Publication (2016) http://dx.doi.org/10.1002/htj.21193
- [11] S. Aberoumand, A. Jafarimoghaddam, K. Javaherdeh, H. Aberoumand; A complete experimental investigation on the rheological behavior of silver- heat transfer oil nanofluid, Heat Transfer – Asian Research, John Wiley & Sons Publication (2016) http://dx.doi.org/10.1002/htj.21212
- [12] A.A. Abbasian Arani, H. Aberoumand, S. Aberoumand, A. Jafari moghaddam, M. Dastanian; An empirical investigation on thermal characteristics and pressure drop of Ag-oil nanofluid in concentric annular tube, Heat and mass transfer, Springer Publication (2015) http://dx.doi.org/10.1007/s00231-015-1686-0
- [13] www.pnf-co.com.
- [14] W.M. Kays, M.E. Crawford, B. Weigand; Convective heat and mass transfer; (fourth ed.)McGrow Hill (2005)
- [15] H.W. Coleman, W.G. Steele; Experimental and Uncertainty Analysis for Engineers; Wiley, New York (1989)
- [16] B. Farajollahi, S.G.H. Etemad, M. Hojjat; Heat transfer of nanofluids in a shell and tube heat exchanger; Int. J. Heat Mass Transfer, 53 (1–3) (2010), pp. 12–17
- [17] D.S. Wen, Y.L. Ding; Effect on heat transfer of particle migration in suspensions of nanoparticles flowing through minichannels; Microfluid Nanofluid, 1 (2) (2005), pp. 183–189
Document information
Published on 12/04/17
Licence: Other
Share this document
Keywords
claim authorship
Are you one of the authors of this document?